Difference between revisions of "Escape Circle"
(Add calculation section) |
m (→See also: add link to Non-iterative PPMEA) |
||
| (9 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| − | '''Escape Circle''' is the circumference of [[Escape Envelope]] assuming the motion of the target is linear and uniform. | + | '''Escape Circle''' is the circumference of [[Escape Envelope]] assuming the motion of the target is linear and uniform, and ignoring any collision with walls or other robots. The "circle" in its name is due to the fact that the shape of the escape envelope in this case is a circle. |
| − | == | + | == Prove & calculation == |
[[File:EscapeCircle.png|600px]] | [[File:EscapeCircle.png|600px]] | ||
| Line 18: | Line 18: | ||
The points defined by (x, y) satisfying above formula is a circle e with center P(-k, 0), and radius sqrt(k<sup>2</sup> + k). | The points defined by (x, y) satisfying above formula is a circle e with center P(-k, 0), and radius sqrt(k<sup>2</sup> + k). | ||
| + | |||
| + | == Example code == | ||
| + | |||
| + | <syntaxhighlight> | ||
| + | import java.awt.geom.Point2D; | ||
| + | |||
| + | public final class EscapeCircle { | ||
| + | public Point2D.Double center; | ||
| + | public double radius; | ||
| + | |||
| + | public EscapeCircle(Point2D.Double center, double radius) { | ||
| + | this.center = center; | ||
| + | this.radius = radius; | ||
| + | } | ||
| + | |||
| + | public static EscapeCircle calc(Point2D.Double fireLocation, Point2D.Double targetLocation, double waveSpeed) { | ||
| + | double distance = fireLocation.distance(targetLocation); | ||
| + | double k = 1. / (sq(waveSpeed / 8.) - 1.); | ||
| + | double radius = distance * Math.sqrt(k * k + k); | ||
| + | |||
| + | return new EscapeCircle(scale(fireLocation, targetLocation, 1 + k), radius); | ||
| + | } | ||
| + | |||
| + | private static Point2D.Double scale(Point2D.Double origin, Point2D.Double point, double ratio) { | ||
| + | return new Point2D.Double( | ||
| + | origin.x + ratio * (point.x - origin.x), | ||
| + | origin.y + ratio * (point.y - origin.y) | ||
| + | ); | ||
| + | } | ||
| + | |||
| + | private static double sq(double x) { | ||
| + | return x * x; | ||
| + | } | ||
| + | } | ||
| + | </syntaxhighlight> | ||
| + | |||
| + | == Relationship with [[Traditional Maximum Escape Angle]] == | ||
| + | |||
| + | Escape circle can be considered as the 2D case of traditional MEA, as [[MEA]] is essentially 1/2 the angle between the tangent rays from the firer to the escape circle, where escape circle can also be derived from the same situation traditional MEA is defined. | ||
| + | |||
| + | With this in mind, one can easily derive the algorithm of [[Non-iterative PPMEA|Non-iterative Precise Positional MEA]] from the definition of escape circle. | ||
== See also == | == See also == | ||
| Line 23: | Line 64: | ||
* [[Escape Envelope]] | * [[Escape Envelope]] | ||
* [[Maximum Escape Angle]] | * [[Maximum Escape Angle]] | ||
| + | * [[Non-iterative PPMEA]] | ||
| + | * [http://web.archive.org/web/20030514212153/http://robocoderepository.com/jive/thread.jsp?forum=18&thread=1052 Shape of the escape envelope] | ||
[[Category:Terminology]] | [[Category:Terminology]] | ||
Latest revision as of 11:16, 8 August 2019
Escape Circle is the circumference of Escape Envelope assuming the motion of the target is linear and uniform, and ignoring any collision with walls or other robots. The "circle" in its name is due to the fact that the shape of the escape envelope in this case is a circle.
Contents
Prove & calculation
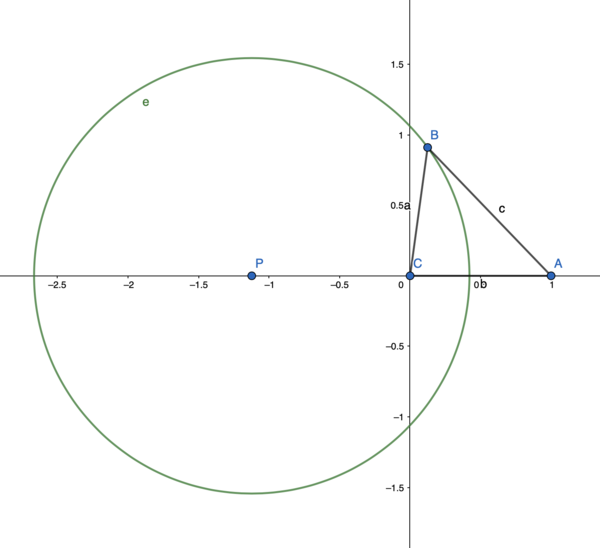
Assuming uniform linear motion, consider a situation where a bullet fired from the firer A hits the target C at some future position B. A is the angle opposite to a, B is opposite to b, and C is opposite to c.
Let the target C be the origin, the distance and orientation from the target to the firer be the unit length and positive x-axis respectively.
Let θ be the angle between b and a, we have c / a = Vb / Vr, b = 1, where Vb and Vr is the velocity of the bullet and the target respectively. Now consider cosine formula:
a2 + 12 - 2 a cosθ = (Vb / Vr a)2
Let x = a cosθ, y = a sinθ, k = 1 / ((Vb / Vr)2 - 1), we have:
(x + k)2 + y2 = k2 + k
The points defined by (x, y) satisfying above formula is a circle e with center P(-k, 0), and radius sqrt(k2 + k).
Example code
import java.awt.geom.Point2D;
public final class EscapeCircle {
public Point2D.Double center;
public double radius;
public EscapeCircle(Point2D.Double center, double radius) {
this.center = center;
this.radius = radius;
}
public static EscapeCircle calc(Point2D.Double fireLocation, Point2D.Double targetLocation, double waveSpeed) {
double distance = fireLocation.distance(targetLocation);
double k = 1. / (sq(waveSpeed / 8.) - 1.);
double radius = distance * Math.sqrt(k * k + k);
return new EscapeCircle(scale(fireLocation, targetLocation, 1 + k), radius);
}
private static Point2D.Double scale(Point2D.Double origin, Point2D.Double point, double ratio) {
return new Point2D.Double(
origin.x + ratio * (point.x - origin.x),
origin.y + ratio * (point.y - origin.y)
);
}
private static double sq(double x) {
return x * x;
}
}Relationship with Traditional Maximum Escape Angle
Escape circle can be considered as the 2D case of traditional MEA, as MEA is essentially 1/2 the angle between the tangent rays from the firer to the escape circle, where escape circle can also be derived from the same situation traditional MEA is defined.
With this in mind, one can easily derive the algorithm of Non-iterative Precise Positional MEA from the definition of escape circle.